This article was published in Scientific American’s former blog network and reflects the views of the author, not necessarily those of Scientific American
I gave my first midterm last week. I'm teaching a roughly junior level class for math majors, one of their first classes that is mostly focused on proofs rather than computations or algorithms. It is more abstract than most math classes they've taken up to this point. I love teaching this class because a class like this one got me excited about math, so it reminds me of a special time in my life.
One of the questions on my midterm was: Describe a set in R2 that is neither open nor closed.
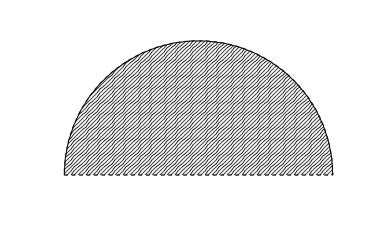
A set that is neither open nor closed. The solid arc on the top of the half circle indicates that part of the boundary is included in the set, while the dotted line at the bottom indicates that part of the boundary is not included in the set. With appropriate labeling and scaling, this set could be described as the set of all points (x,y) that are less than or equal to 1 unit away from the point (0,0) and have a y-coordinate that is strictly greater than 0. Credit: Evelyn Lamb.
On supporting science journalism
If you're enjoying this article, consider supporting our award-winning journalism by subscribing. By purchasing a subscription you are helping to ensure the future of impactful stories about the discoveries and ideas shaping our world today.
"Open" and "closed" are, of course, technical terms. In our class, a set is called "open" if around every point in the set, there is a small ball that is also contained entirely within the set. If we just look at the real number line, the interval (0,1)—the set of all numbers strictly greater than 0 and strictly less than 1—is an open set. If you pick some number in the interval (0,1), no matter how close it is to one of the endpoints, there is some smaller interval around it that is also entirely contained in the interval (0,1). For example, the number 1/100 is very close to 0, but the interval (1/200, 1/50) contains the point 1/100 and is entirely contained in the interval (0,1). On the other hand, the interval [0,1]—the set of all numbers greater than or equal to 0 and less than or equal to 1—is not open. The only difference between [0,1] and (0,1) is whether we include the endpoints, but those two little points make a big difference. Every interval around the point 0 contains negative numbers, so there is no little interval around the point 0 that is entirely in the interval [0,1]. Hence the interval [0,1] doesn't satisfy the definition of open. (For more on open sets, check out Wikipedia or MathWorld.)
A set is called "closed" if its complement is open. Our class takes place almost entirely in normal Euclidean space, rather than some more exotic space. In d-dimensional Euclidean space Rd, the complement of a set A is everything that is in Rd but not in A. The interval [0,1] is closed because its complement, the set of real numbers strictly less than 0 or strictly greater than 1, is open.
So the question on my midterm exam asked students to find a set that was not open and whose complement was also not open. I thought this was going to be one of the easier questions on the exam, so I was surprised that many of my students made the same mistake on it. Instead of giving me sets that were neither open nor closed, they gave me sets that were both open and closed!
I had underestimated the power of the English language to suggest mathematically incorrect statements to my students. In mathematics, "open" and "closed" are not antonyms. Sets can be open, closed, both, or neither. (A set that is both open and closed is sometimes called "clopen.") The definition of "closed" involves some amount of "opposite-ness," in that the complement of a set is kind of its "opposite," but closed and open themselves are not opposites. If a set is not open, that doesn't make it closed, and if a set is closed, that doesn't mean it can't be open. They're related, but it's not a mutually exclusive relationship. But in English, the two words are basically opposites (although for doors and lids, we have the option of "ajar" in addition to open and closed). My students used their intuition about the way the words "open" and "closed" relate to each other in English and applied that intuition to the mathematical use of the terms.
Some of them even justified their answers by saying something along the lines of "because [A] is open, it is not closed, and because it is closed, it is not open." They knew factually that there was a set that was both open and closed, but they didn't quite grok it, so they had somehow come to the self-contradictory conclusion that a set that was both open and closed was neither open nor closed!
There were of course other factors besides language at play here: time can be an issue in an exam setting, and the pressure of taking tests sometimes makes people write bizarre things that even they don't understand later. But I think the differences between the mathematical and English meanings of the words "open" and "closed" played a large factor in my students' difficulty with the exam question. I don't think they would have made the same mistake about pizza toppings. This pizza has both cheese and pepperoni on it. Cheese is not pepperoni, and pepperoni is not cheese, so this pizza has "not cheese" and "not pepperoni" on it and hence it has neither cheese nor pepperoni. "Pepperoni" and "cheese" are not opposites in English the way "closed" and "open" are.
My students' mistakes on this question were valuable for me and I hope for them as well, despite the lost points. I learned that my students are still getting used to the concepts of "open" and "closed," which will continue to be important in the rest of the class, and more importantly that they're still getting used to working with mathematical definitions. I think mathematicians are unusually good at accepting a new definition, ignoring prior knowledge, and just working with the definition. We poke at different parts of the definition and try to see how it would be different if we removed or added clauses. What kinds of theorems can we get "for free" from a definition? Creating good definitions is an art, as Cathy O'Neil discusses here, and it's very important in mathematics. But my students are brand new mathematicians, and they aren't skilled in this art yet.
There is a blog called Math Mistakes that collects interesting examples of incorrect middle- and high-school student work and analyzes it. What are students thinking when they make these mistakes? What is the best way to address their misunderstandings? Contrary to popular belief, exams are not strictly torture devices or tools of punishment. An exam can also be a way to asses student progress and diagnose student misconceptions. I hope that now that I have diagnosed a common misunderstanding of "open" and "closed" in my class, I can clear it up and try to avoid similar errors in the future.