Gedankenexperiment, German for “thought experiment,” was Albert Einstein’s famous name for the imaginings that led to his greatest breakthroughs in physics. He traced his realization of light’s finite speed—the core idea of special relativity—to his teenage daydreams about riding beams of light. General relativity, his monumental theory of gravitation, has its origins in his musings about riding up and down in an elevator. In both cases, Einstein crafted new theories about the natural world by using his mind’s eye to push beyond the limitations of laboratory measurements.
Einstein was neither the first nor the last theorist to do this, but his remarkable achievements were pivotal in establishing the gedankenexperiment as a cornerstone of modern theoretical physics. Today physicists regularly use thought experiments to formulate new theories and to seek out inconsistencies or novel effects within existing ones.
But the modern embrace of thought experiments raises some uncomfortable questions. In the search for a grand unified theory that would wed the small-scale world of quantum mechanics with Einstein’s relativistic description of the universe at large, the most popular current ideas are bereft of observational support from actual experiments. Can thought alone sustain them? How far can we trust logical deduction? Where is the line between scientific intuition and fantasy? Einstein’s legacy offers no certain answers: On one hand, his reliance on the power of thought was a spectacular success. On the other, many of his best-known thought experiments were based on data from real experimentation, such as the classic Michelson-Morley experiment that first measured the constancy of the speed of light. Moreover, Einstein’s fixation on that which can be measured at times blinded him to deeper layers of reality—although even his mistakes in thought experiments contributed to later breakthroughs.
On supporting science journalism
If you're enjoying this article, consider supporting our award-winning journalism by subscribing. By purchasing a subscription you are helping to ensure the future of impactful stories about the discoveries and ideas shaping our world today.
Here we will walk through some of Einstein’s most iconic thought experiments, highlighting how they succeeded, where they failed and how they remain vital to questions now at the frontiers of theoretical physics.
The Windowless Elevator
In his thought experiments, Einstein’s genius was in realizing which aspects of experience were essential and which could be discarded. Consider his most famous one: the elevator thought experiment, which he began devising in 1907. Einstein argued that inside a windowless elevator, a person cannot tell whether the elevator is at rest in a gravitational field or is instead being hauled up with constant acceleration. He then conjectured that the laws of physics themselves must be identical in both situations. According to this “principle of equivalence,” locally (in the elevator), the effects of gravitation are the same as those of acceleration in the absence of gravity. Converted into mathematical equations, this principle became the basis for general relativity. In other words, the elevator thought experiment motivated Einstein to make the daring intellectual leap that ultimately led to his greatest achievement, his geometric description of gravity.

Credit: Nigel Holmes
Spooky Action
Later in his career, Einstein fought hard against the tenets of quantum mechanics, particularly the uncertainty principle, which dictates that the more you know about one aspect of a fundamental particle, such as its position, the less you can know about another, related aspect of that particle, such as its momentum—and vice versa. Einstein thought that the uncertainty principle was a sign that quantum theory was deeply flawed.
During a years-long exchange with Danish quantum theorist Niels Bohr, Einstein conceived of a series of thought experiments meant to demonstrate that it is possible to violate the uncertainty principle, but Bohr dissected every one of them. This exchange bolstered Bohr’s conviction that quantum uncertainty was a fundamental aspect of nature. If not even the great Einstein could devise a way to precisely measure both the position and the momentum of a particle, then certainly there must be something to the uncertainty principle!
In 1935, along with his colleagues Boris Podolsky and Nathan Rosen, Einstein published what was meant to be his most potent critique of the uncertainty principle. Perhaps because Podolsky, not Einstein, drafted the actual text of the paper, this Einstein-Podolsky-Rosen (EPR) thought experiment was presented not as an easy-to-imagine scenario of boxes, clocks and light beams but as an abstract series of equations describing interactions between two generalized quantum systems.
The simplest version of the EPR experiment studies the paradoxical behavior of “entangled” particles—pairs of particles that share a common quantum state. It unfolds as follows: Imagine an unstable particle with a spin of zero decaying into two daughter particles, which speed off in opposite directions. (Spin is a measure of a particle’s angular momentum, but counterintuitively, it has little to do with a particle’s rate of rotation.) Conservation laws dictate that the spins of those two daughter particles must add up to zero; one particle, then, could possess a spin value of “up,” and the other could have a spin value of “down.” The laws of quantum mechanics dictate that in the absence of measurement, neither of the particles possesses a definite spin until one of the two speeding entangled particles is measured. Once a measurement of one particle is made, the state of the other changes instantaneously, even if the particles are separated by vast distances!
Einstein believed this “spooky action at a distance” was nonsense. His own special theory of relativity held that nothing could travel faster than light, so there was no way for two particles to communicate with each other instantaneously from opposite sides of the universe. He suggested instead that the measurement outcomes must be determined prior to measurement by “hidden variables” that quantum mechanics failed to account for. Decades of discussion followed until 1964, when physicist John Stewart Bell developed a theorem quantifying exactly how the information shared between entangled particles differs from the information that Einstein postulated would be shared through hidden variables.
Since the 1970s lab experiments with entangled quantum systems have repeatedly confirmed that Einstein was wrong, that quantum particles indeed share mutual information that cannot be accounted for by hidden variables. Spooky action at a distance is real, but experiments have demonstrated that it cannot be used to transmit information faster than light, making it perfectly consistent with Einstein’s special relativity. This counterintuitive truth remains one of the most mysterious conundrums in all of physics, and it was Einstein’s stubborn, mistaken opposition that proved crucial to confirming it.
Alice and Bob
Today some of the most significant thought experiments in physics explore how to reconcile Einstein’s clockwork, relativistic universe with the fuzzy uncertainties inherent to quantum particles.
Consider, for instance, the widely discussed black hole information paradox. If you combine general relativity and quantum field theory, then you find that black holes evaporate, slowly radiating away their mass because of quantum effects. You also find that this process is not reversible: regardless of what formed the black hole, the evaporating black hole always produces the same featureless bath of radiation from which no information about its contents can be retrieved. But such a process is prohibited in quantum theory, which states that any occurrence can, in principle, be reversed in time. For instance, according to the laws of quantum mechanics, the leftovers of a burned book still contain all the information necessary to reassemble that book even though this information is not easily accessible. Not so for evaporating black holes. And so we arrive at a paradox, a logical inconsistency. A union of quantum mechanics and general relativity tells us that black holes must evaporate, but we conclude that the result is incompatible with quantum mechanics. We must be making some mistake—but where?
The thought experiments created to explore this paradox typically ask us to imagine a pair of observers, Bob and Alice, who share a pair of entangled particles—those spooky entities from the EPR experiment. Alice jumps into the black hole, carrying her particle with her, whereas Bob stays outside and far away with his. Without Alice, Bob’s particle is just typical, with a spin that might measure up or down—the information that it once shared with its entangled partner is lost, along with Alice.
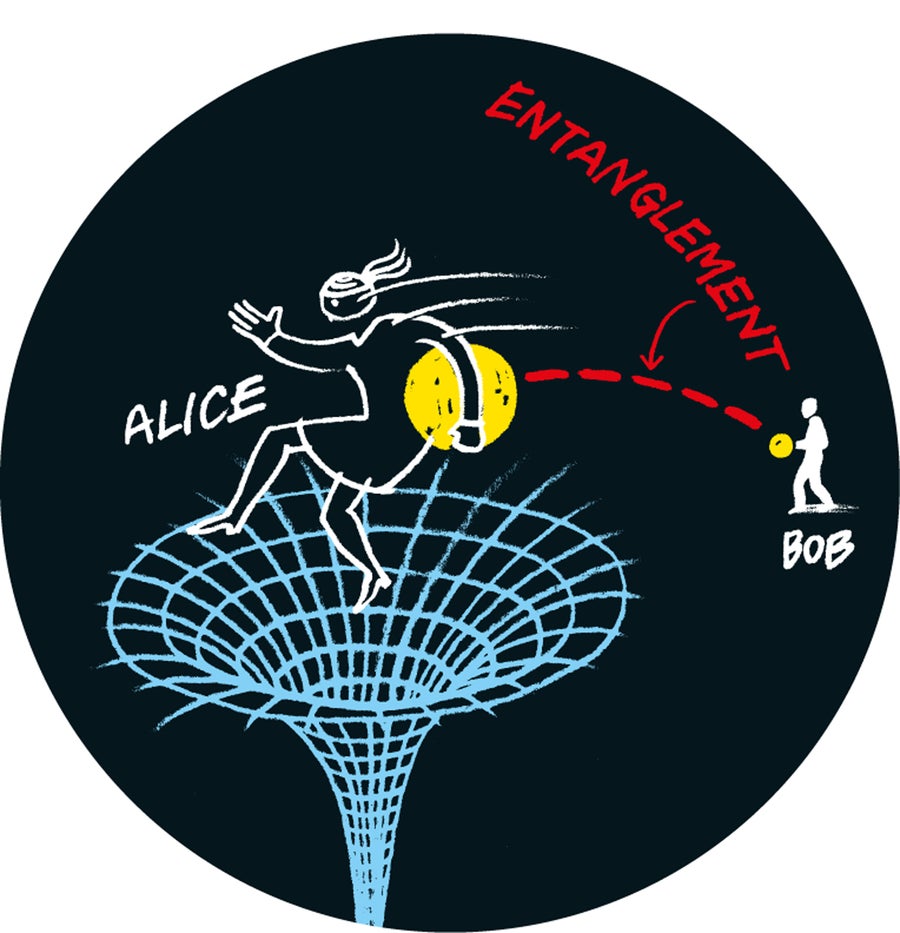
Credit: Nigel Holmes
Bob and Alice play a central role in one of the most popular proposed solutions to the paradox, called black hole complementarity. Proposed in 1993 by Leonard Susskind, Lárus Thorlacius and John Uglum, all then at Stanford University, black hole complementarity rests on Einstein’s golden rule for a gedankenexperiment: a strict focus on that which can be measured. Susskind and his colleagues postulated that the information falling in with Alice must come out later with the evaporating black hole’s radiation. This scenario would usually create another inconsistency because quantum mechanics allows only pair-wise entanglement with one partner at a time, a property called monogamy of entanglement. That is, if Bob’s particle is entangled with Alice’s, it cannot be entangled with anything else. But black hole complementarity requires that Bob’s particle be entangled with Alice’s and with the radiation the black hole later emits even though this violates monogamy. At first sight, then, black hole complementarity seems to exchange one inconsistency with another.
But like a perfect crime, if no one actually witnesses this inconsistency, perhaps it can subvert nature’s otherwise strict laws. Black hole complementarity relies on the argument that it is physically impossible for any observer to see Alice and Bob’s entangled particles breaking the rules.
To envision how this perfect quantum-mechanical crime could unfold, imagine a third observer, Charlie, hovering near the black hole, keeping an eye on Alice and Bob. He watches as Bob stays outside and as Alice falls in, measuring the black hole’s emitted radiation all the while. In theory, information encoded in that radiation could tip off Charlie that Bob and Alice had violated the monogamy of their entanglement. To know for certain, however, Charlie would have to compare his observations not only with Bob’s measurement but also with Alice’s— inside the black hole. So he must hover at the horizon, measure the emitted radiation, then jump in to tell Alice what he has found. Amazingly enough, Susskind and Thorlacius showed that no matter how hard Charlie tries, it is impossible for him to enter the black hole and compare his information with Alice’s before they are both torn apart by tidal forces. Their grisly fate suggests no violations of quantum mechanics can ever be measured by anybody around a black hole, and so theorists can commit this crime against nature with impunity.
.jpg?w=900)
Credit: Nigel Holmes
Suffice it to say, not all theorists are convinced that this argument is valid. One criticism of black hole complementarity is that it might violate Einstein’s equivalence principle—the one that grew out of his elevator thought experiment. Einstein’s general relativity predicts that just as the elevator’s passenger cannot distinguish between gravity and acceleration, an observer crossing a black hole’s horizon should not notice anything unusual; there is no way an observer can tell that he or she has slipped past the point of no return.
Now let us return to the entanglement of Alice and Bob. If the radiation that Bob sees from far outside the hole contains all the information that we thought vanished with Alice behind the horizon, then this radiation must have been emitted with an extremely high energy; otherwise it would not have escaped the strong gravitational pull near the horizon. This energy is high enough to vaporize any infalling observer before he or she slips past the black hole’s horizon. In other words, black hole complementarity implies that black holes have a “firewall” just outside the horizon—and yet the firewall directly contradicts the predictions of Einstein’s equivalence principle.
At this point, we have ventured deep into the realm of theory. Indeed, we might never know the solutions to these puzzles. But because those solutions could lead to an understanding of the quantum nature of space and time, these puzzles are, for better or worse, some of the most vibrant areas of research in theoretical physics. And it all goes back to Einstein’s musings about falling elevators.
